No products in the cart.
Categories
My Publication
Shopping cart (0)
Subtotal: ₹0.00
Spend ₹2,000.00 to get free shipping
Congratulations! You've got free shipping.
₹425.00 Original price was: ₹425.00.₹415.00Current price is: ₹415.00.
Writers:
- Dr. Harikishan (K.R. {P.G.} College, Mathura)
- Dr. Vidya Sagar Chaubey (B.R.D. {P.G.} College, Deoria)
- Dr. Vijai Shankar Verma (D.D.U. Gorakhpur University, Gorakhpur)
- Dr. Prateek Mishra (M.L.K.{P.G.} College, Balrampur)
21 Students are viewing this books right now
🚚 Shipping Rates & Delivery Dates: See at Checkout!
शिपिंग दरें और डिलीवरी की तारीखें: चेकआउट पर देखें!
🎉 Special Offers on Books: Explore at Checkout!
📚 किताबों पर विशेष ऑफर: चेकआउट पर देखें!
💳 Pay Prepaid & Get Extra 5% Off!
प्रीपेड भुगतान करें और अतिरिक्त 5% छूट पाएं!
🛒 Shop All Books Now!
🛒 सभी किताबें अभी खरीदें!
🚚 Shipping Rates & Delivery Dates: See at Checkout!
शिपिंग दरें और डिलीवरी की तारीखें: चेकआउट पर देखें!
🎉 Special Offers on Books: Explore at Checkout!
📚 किताबों पर विशेष ऑफर: चेकआउट पर देखें!
💳 Pay Prepaid & Get Extra 5% Off!
प्रीपेड भुगतान करें और अतिरिक्त 5% छूट पाएं!
🛒 Shop All Books Now!
🛒 सभी किताबें अभी खरीदें!
Your Payment is 100% Secure
| Weight | 0.500 kg |
|---|---|
| Dimensions | 15.00 × 1.5 × 24.00 cm |
| Pub.of Book | Ramprasad Pub. Agra |
| Sub. Of Book | B.Sc. 1st Sem. |
| Subject Of Book | Mathematics Part-A |
| No. Of Pages | 480 Pg |
Publication: Ramprasad Publication, Agra
Writers:
- Dr. Harikishan (K.R. {P.G.} College, Mathura)
- Dr. Vidya Sagar Chaubey (B.R.D. {P.G.} College, Deoria)
- Dr. Vijai Shankar Verma (D.D.U. Gorakhpur University, Gorakhpur)
- Dr. Prateek Mishra (M.L.K.{P.G.} College, Balrampur)
Unit I: Foundations and Series
- Indian Ancient Mathematics and Mathematicians
- A brief introduction to the contributions of ancient Indian mathematicians.
- Sequences of Real Numbers
- Definition of a sequence.
- Theorems on the limits of sequences.
- Bounded and monotonic sequences.
- Cauchy’s convergence criterion and Cauchy sequence.
- Limit superior and limit inferior of a sequence.
- Subsequence.
- Infinite Series
- Series of non-negative terms.
- Convergence and divergence tests.
- Comparison tests, Cauchy’s integral test.
- Ratio and root tests.
- Raabe’s logarithmic test.
- de Morgan and Bertrand’s tests.
- Alternating Series
- Alternating series and Leibnitz’s theorem.
- Absolute and conditional convergence.
Unit II: Limits, Continuity, and Differentiability
- Limit, Continuity, and Differentiability of Function
- Definitions of limit, continuity, and differentiability of a function of a single variable.
- Cauchy’s and Heine’s definitions.
- Equivalence of Cauchy’s and Heine’s definitions.
- Uniform continuity.
- Theorems: Borel’s, Boundedness, Bolzano’s, Intermediate value, and Extreme value.
- Darboux’s Intermediate Value Theorem for Derivatives and Chain Rule
- Explanation of Darboux’s theorem.
- Application of the chain rule in differentiation.
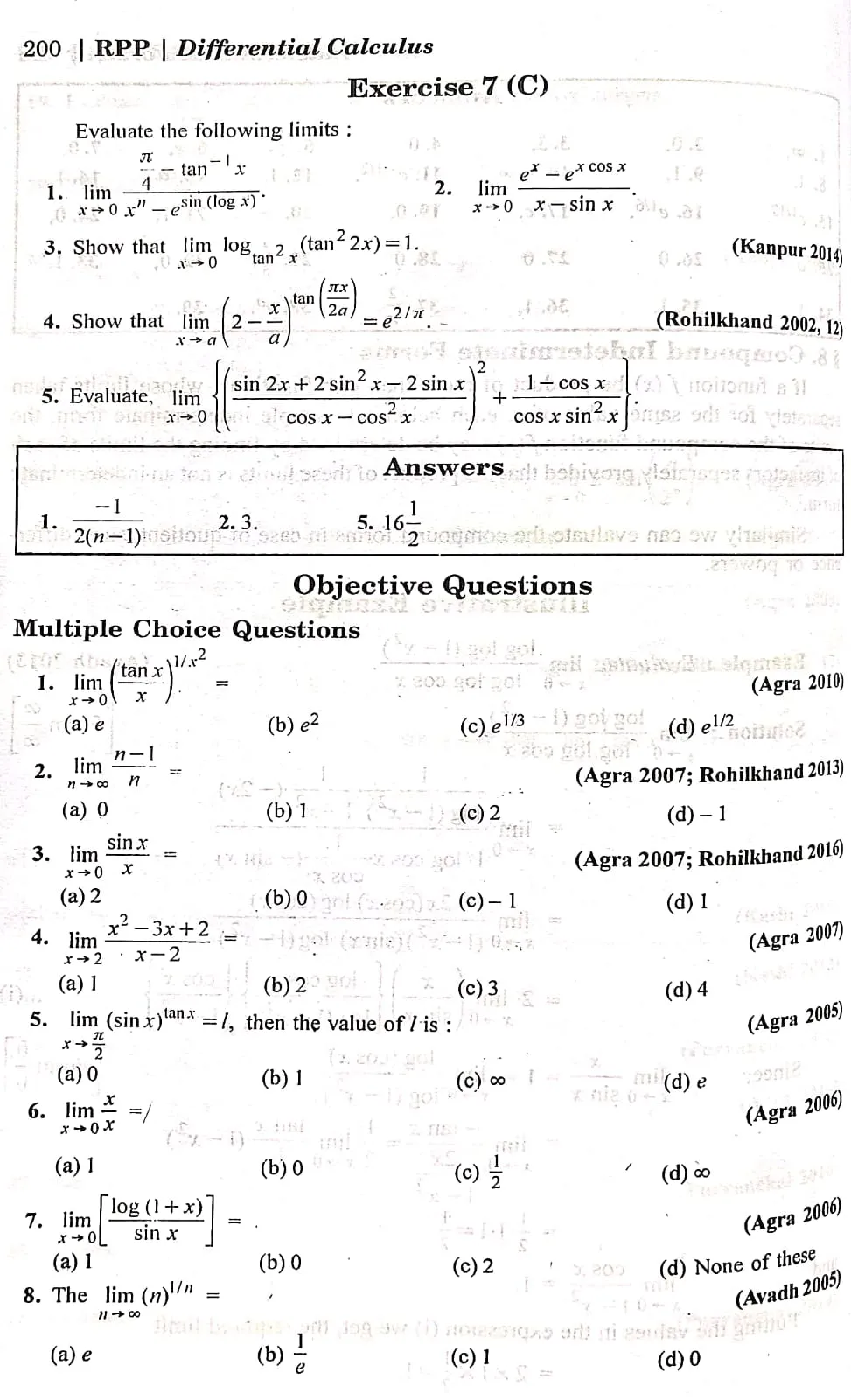
- Indeterminate Forms
- Resolution and evaluation of indeterminate forms.
Unit III: Theorems and Series Expansions
- Rolle’s Theorem, Lagrange’s and Cauchy Mean Value Theorems, Higher Order Mean Value Theorems
- Detailed discussion of Rolle’s, Lagrange’s, and Cauchy’s theorems.
- Introduction to the Mean Value Theorem of higher orders.
- Taylor’s Theorem with different forms of remainders.
- Successive Differentiation and Leibnitz’s Theorem
- Successive differentiation of functions.
- Leibnitz’s theorem for the nth derivative of a product.
- Maclaurin’s and Taylor’s Series
- Expansion of functions using Maclaurin’s and Taylor’s series.
- Partial Differentiation and Euler’s Theorem on Homogeneous Functions
- Introduction to partial differentiation.
- Euler’s theorem for homogeneous functions and its applications.
Unit IV: Geometry of Curves
- Tangents and Normals
- Calculation and interpretation of tangents and normals to curves.
- Asymptotes
- Identifying and calculating asymptotes for curves.
- Curvature
- Definition and calculation of curvature.
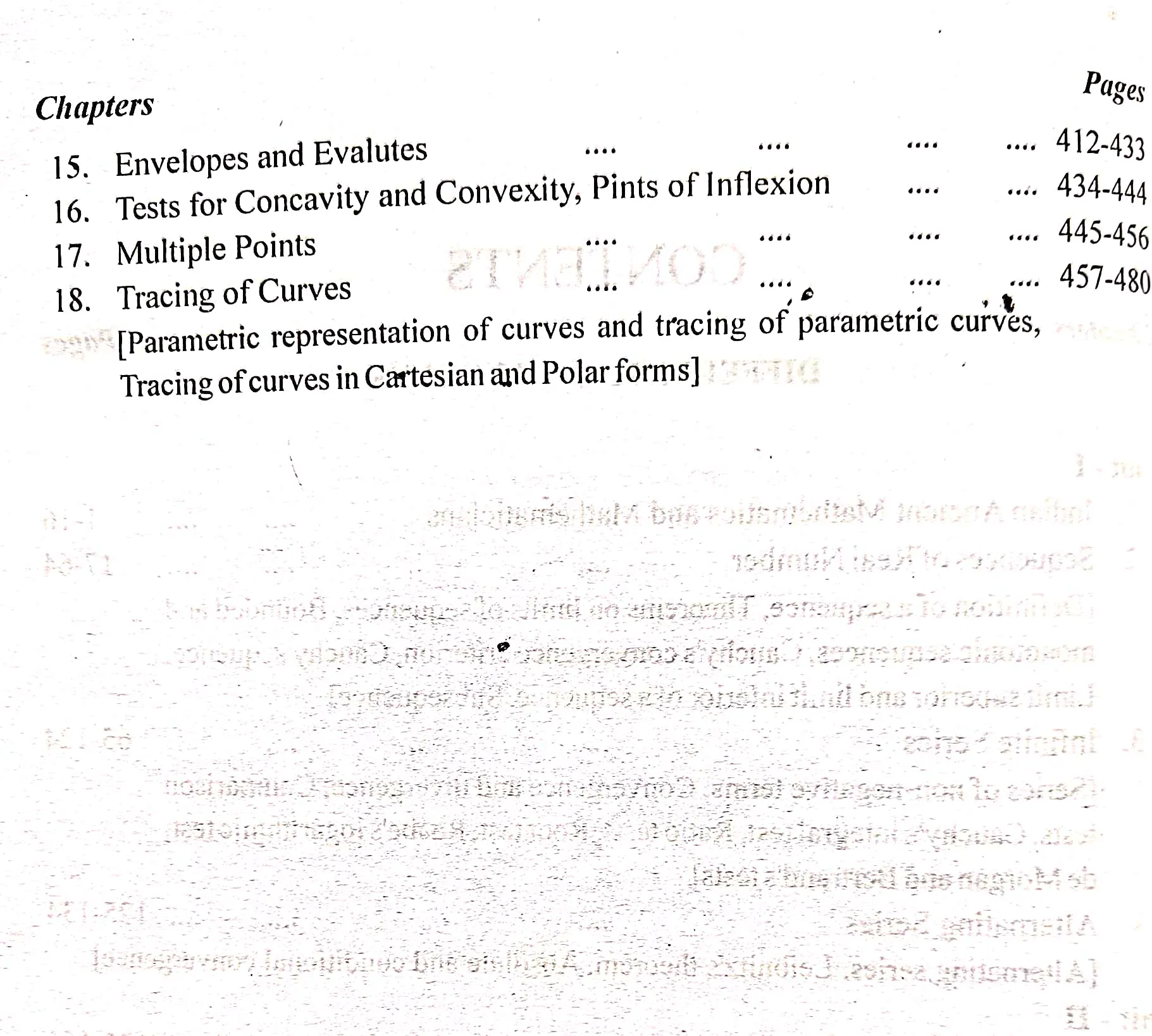
- Envelopes and Evolutes
- Concepts of envelopes and evolutes, their derivation, and properties.
- Tests for Concavity and Convexity, Points of Inflexion
- Methods for testing concavity and convexity.
- Identifying points of inflexion in curves.
- Multiple Points
- Analysis of multiple points on a curve.
- Tracing of Curves
- Parametric representation of curves and their tracing.
- Techniques for tracing curves in Cartesian and polar coordinates.
This book is designed to introduce students to the core concepts of Differential Calculus, with a focus on the application of calculus in both theoretical and practical problems. Each unit methodically builds the student’s understanding of sequences, series, differentiation, and curve tracing, incorporating tests and theorems essential for deeper exploration into higher-level mathematics.
Be the first to review “Differential Calculus Rpp” Cancel reply
Related products
Sale
Chemistry-5th Sem. Rearrangements &Chemistry Of Group Elements Paper-2nd Pragati
🔥 2 items sold in last 7 days
Sale
Quantum Mechanics And Spectroscopy Ramprasad
🔥 7 items sold in last 7 days
Sale
Diversity Of Chordates And Comparative Anatomy “Zoology” B.Sc. 5th Sem. Ramprasad
🔥 5 items sold in last 7 days
Sale
Plant Physiology Metabolism And Biochemistry Botany B.Sc. 5th Sem Ramprasad
🔥 4 items sold in last 7 days
Sale
Integral Calculus &Vector Calculus Rpp
🔥 5 items sold in last 7 days
You may add any content here from XStore Control Panel->Sales booster->Request a quote->Ask a question notification
At sem a enim eu vulputate nullam convallis Iaculis vitae odio faucibus adipiscing urna.
























Reviews
There are no reviews yet.